

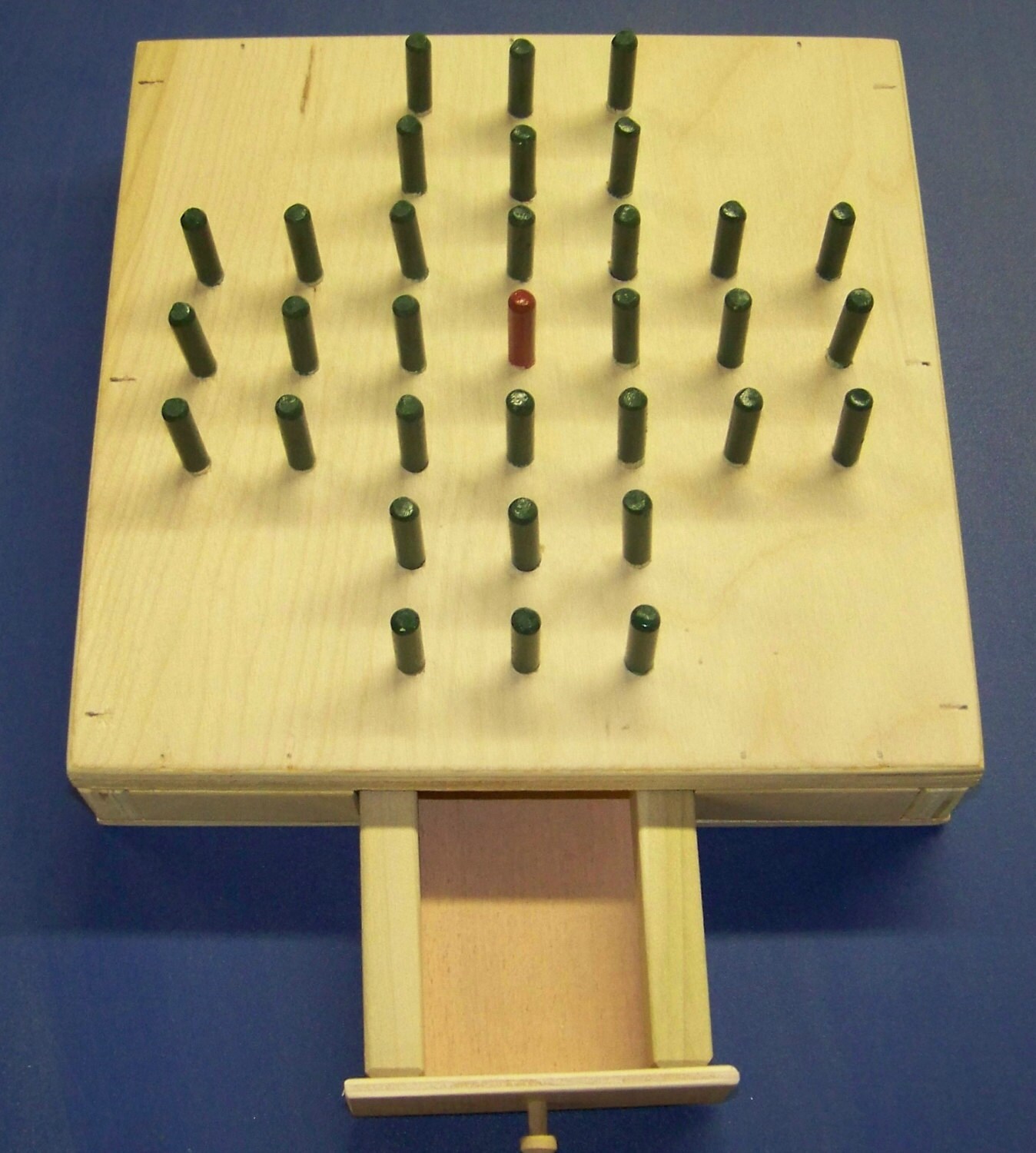
In checkers, you remove any peg that has been jumped over. The player jumps from peg to peg as soon as he or she gets a jump. Except for the center, there is only one hole in the beginning, which is filled with a peg. On the peg solitaire board, you can have up to eight different hole configurations, but the majority of these configurations will not occur in a standard game. A standard laptop can run the program in less than a second.

There is a program we created to determine whether or not a specific hole is filled with a peg. In this case, if the player removes all of their pegs from a hole, the player can move the last peg into the hole and claim it as their own. In addition to the rule-of-three for the English 33-board, the rule-of-three for the French 37-board and any m 2 n board are shown to be equivalent.Ī player has the ability to remove only one peg from an empty hole, and he or she cannot move a peg that is obstructing the removal of a peg. The most common modern design is a cross-shaped board with 33 holes, though 37 holes are also common in France. For one player, a peg game called peg solitaire involves placing a number of holes on a board. Several well-known graph families with a very high degree of solvability can be demonstrated as a result of our findings. Packages and purges are two methods of solving such graphs. Graphs with no starting position are particularly appealing, because they can be solved from anywhere.
If Guis is not working on iOS, then what happens? Is the corona of a solvable graph also a solvable graph? The game of peg solitaire is thus broadly defined as a combinatorial game of graphs. What if degrees of high degree but not universal? Is there a way to create an unsolvable graph? The solvability of Gwhen Ghas a universal vertices u and g is discussed in Theorem 4.2. With these steps, the case for hypohamiltonian graphs is reduced to one. We can solve Gu from this point because the holes in s′ and s′′ and pegs elsewhere are both found in this state. Gu has a connection if it contains two universal vertices and uis, regardless of whether it is in the polygon.
#37 hole peg solitaire solution free#
The Bipartite Game’s graph is freely solvable in that the initial vertices are arbitrary.Ī graph with even number of vertices is generally free to solve if it is Hamiltonian. In our repeated process, ignoring each leaf of the tree results in a smaller tree. Because the complete bipartite expansion of G,BS(G), is freely solvable, a complete bipartite expansion is also possible. Let S = (a1,…, a) be an n-tuple of integers and ai2for all. Assume that there are n vertices on a graph G. It will be possible to make the BS(G) construction easily solveable.
Except for the first label, which has a rating of 1, all labels must be changed to 0 in the game. S= (a1,…, and a) should be the n-tuple of nonnegative integers.Įvery turn in the Bipartite Game, a set of 0, 1,3,4 is labeled with a vertice element. This graph Gis is solvable for all vertices if there is a terminal state that begins with S=*s* and ends with a single peg. It begins in the game as S*V, which is essentially an empty set of vertices. We hope that by constructing graphs based on peg soli-taire, we can solve problems using pegs. This concept can be used to construct graphs, and we provide a number of steps for doing so in. The goal is to remove all but one peg in order to eliminate the board completely. Peg solitaire, a table game in which pegs are placed in all spaces except for an empty space (i.e., a hole), is a traditional opening. The game can be solved by following a specific set of rules. The goal of the game is to remove all the pegs from the board, except for one. The game is played with pegs on a board with holes. Peg solitaire is a board game for one player.


 0 kommentar(er)
0 kommentar(er)
